Schon durch den Titel ist klar, dass nun kein Artikel der Krennwurzn kommen kann, sondern nur eine kleine Einleitung zu einer hochinteressanten Endspielanalyse. Vor ein paar Jahren trat der ehemalige Vereinskollege und Schachfreund FM Heinrich Rolletschek an die Krennwurzn heran und erzählte ihr, dass er einen Fehler in einer bedeutenden Endspielanalyse von GM John Nunn gefunden hat und fragte die Krennwurzn, ob sie bereit wäre seine Analysen mit dem Computer zu überprüfen.
Nun(n) muss man dazu dem Leser noch erklären, dass FM Heinrich Rolletschek so etwas wie ein „Alpen-John-Nunn“ ist und in unserem Lande seit vielen Jahren eine Institution in Endspielfragen ist. Ebenso wie GM John Nunn und der Deutsche GM Karsten Müller ist FM Heinrich Rolletschek ein begnadeter Mathematiker, hat aber im Gegensatz zu den beiden vorgenannten die Mathematik und nicht Schach zu seinem Brotberuf gemacht und unterrichtet als Professor am RISC (Research Institute for Symbolic Computation) der Johannes Kepler Universität in Hagenberg bei Linz.
Aber verlassen wir das Geschwafel der Krennwurzn und wechseln einmal die Sprache, denn auch ein Brexit sollte nicht zu einem Engxit führen und uns von der Sprache der Wissenschaft entfremden.
Analysis of a bishop ending
by FM Dr. Heinrich Rolletschek, Austria
Diagram 1 shows a position from the game Pinter–Alterman, Beersheba 1991, which had previously been analyzed by the winner, Pinter, in Chess Informant, in Nunn’s Chess Endings, and in Fundamental Chess Endings by Mu?ller und Lamprecht. All these authors conclude that Black could have drawn with correct defense. The goal of this article is to show that Position 1 is already winning for White. In fact White always wins with the white king on d4, the black king on d6 and bishops on white squares, unless of course Black to move can capture the white bishop. (In Diagram 1 the kings will immediately move to d4 and d6, respectively.)
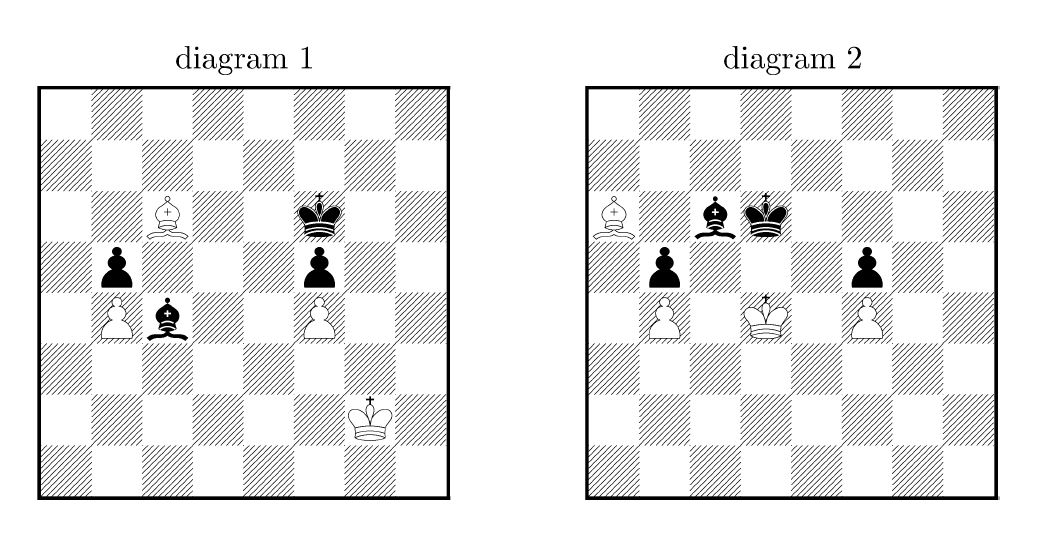
As preceding analysis by Nunn and others shows, White wins if he manages to get his bishop to the central square d5; in this case Black cannot prevent the loss of a pawn, no matter where his bishop is placed. Black’s best defence is to answer the move Bd5 by Bd7, reaching Diagram 4. In the game Alterman resigned when the loss of a pawn was imminent, and Nunn says nothing as to how White should go about realizing the extra pawn. Indeed, at this point it is justified to assess the game as won for White on general grounds; after all, the pawns are sufficiently far apart, and Black’s remaining pawn is still blocked on a white square. Even so, it seems that the win is still not entirely trivial and that it is worthwhile investigating how play may continue. (Of course, this ending is also covered by the recently constructed tablebase for seven-piece endgames.)
Consider Diagram 5. (If Black gives up the Pb5 rather than Pf5, the winning procedure is similar.) We will describe a winning method which is certainly not the only one but absolutely reliable. White starts with the move f5, then moves the king towards g5 and threatens to support the passed pawn. This forces the black king to move in front of the Pf5, allowing White to break through to the pawn b5 and reach a position akin to Diagram 6. Here White still has to work a little bit, since 1. B×b5? B×f5 does not win. However, after an appropriate preparation a pawn exchange does win. As a matter of fact, the main variation starting from Diagram 2 does not lead to Diagram 6, but to a related position which is reached from Diagram 6 after the moves 1. Bc2 Be8 2. Bb1 Bd7 3. Bd3 Kf6 4. Kd5 Ke7
5. Be4.
The preceding discussion indicates that the winning procedure in Position 2 consists of the following five stages:
Stage 1: White gets the bishop out of the upper left corner region and reaches Position 3, first with White to move.
Stage 2: White gets the bishop to the central square d5. (This is the intermediate goal which, according to earlier authors, White is unable to accomplish.)
Stage 3: White forces the win of a pawn, typically after reaching Position 3 with Black to move.
Stage 4: After winning the Pf5, White makes a bypass manoevre, threatening to support the passed pawn, and thus forces a breakthrough to the Pb5.
Stage 5: White exchanges the Pf5 for Black’s Pb5 such that a winning ending B+P v. B is reached.
Stage 3 is dealt with in detail by previous authors, and there is no need to repeat their analysis; we will give only one main line for this part of the winning procedure. Stages 1 and 4 are fairly straightforward, and may be dealt with without considering many different lines; it is not hard to establish that White can always achieve the respective intermediate goals. Although Stage 5 is no particularly complicated either, it is slightly tricky and involves the kind of play where concrete and precise analysis is called for.
Not surprisingly, the central part of our analysis concerns Stage 2. As noted, previous authors claim that White cannot achieve the specified intermediate goal, so what about it? Nunn writes:
You will often find attempts in endgame books to analyse such positions based on the kind of corresponding-squares analysis we saw in [ . . . ]. In this type of analysis, the various positions of the bishops are examined to see which form reciprocal zugzwangs. The trouble with this method is that play is often not restricted to bishop manoeuvres; for example, in [ . . . ] a triangulation by the
whithe king played a crucial role in one line and without this White would not be able to win. Therefore the assumption that king manoeuvres will play no part may not be justified and can give rise to incorrect conclusions.
With this remark Nunn refers to his analysis of Stage 3, but, as it turns out, it also provides the hint for Stage 2! For this stage earlier analysis did not consider a temporary retreat of the white king, but with bishop manoeuvres alone White is indeed unable to achieve his goal. We note in passing that king manoevres occur in each of the five stages, but Stages 2 and 3 are the only ones where this is not obvious in the first place.
At first glance it may seem surprising that a temporary king retreat is the key to White’s success during Stage 2. Given that bishop manoeuvres are not sufficient, it may appear that Black can defend himself by the following strategy:
- answer bishop moves by bishop moves as if the kings remained on d4 and d6;
- answer a retreat of the white king by a retreat of the black king;
- when the white king moves in the background, then move the black king in the background while keeping contact with the square d6;
- when the white king moves back to d4, then move the black king back to d6.
However, this defence strategy fails for a subtle reason. The point is that whenever the black king moves to a white square, it causes some damage to the defence: sometimes the black bishop is obstructed, and sometimes the black king is exposed to a bishop check, which may likewise upset the balance. On the other hand, if the black king only moves on black squares, then he can return to d6 only in an even number of moves, and this allows White to apply triangulation.
In spite of the above remark by Nunn, we feel that it makes sense to consider corresponding squares in this ending, as applicable for Stage 2. Of course, the meaning of corresponding squares has to be defined appropriately; they are characterized as follows. Suppose that the white king is on d4, the black king on d6, the white bishop on a square X, and it is White to move. Then the following assertions hold:
(1) If the black bishop is not standing on one of the squares corresponding to X, then White can at least finish Stage 2 with bishop moves alone; in some cases White can even directly win a pawn or reach some position which occurs during Stage 3 in the main variation.
(2) If the black bishop is standing on one of the squares corresponding to X, then White cannot achieve any of the goals specified under (1) without a temporary retreat of the king.
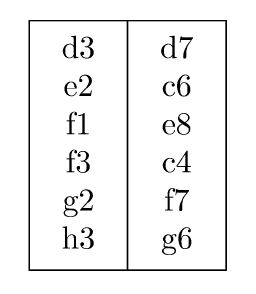
With kings on d4 and d6, it is always Black’s best defence to move his bishop to a corresponding square. Similarly, with kings one move away from d4 and d6, respectively (and not on d5), Black should either move the bishop to a corresponding square or else play Kd6, otherwise White achieves his goal after playing Kd4 and forcing Kd6. Thus knowledge of corresponding squares provides valuable help for the analysis. Moreover, we may immediately stop analysis in any secondary line as soon as a position is reached with kings on d4 and d6, White to move and Black’s bishop not on a corresponding square, provided that assertion (1) above has been established for the case in question. The following table shows the most the most important pairs of corresponding squares:
During Stage 2 the white bishop will not move to any square other than d3, e2, f1, f3, g2 and h3 until d5 is occupied, except for one line where a pawn is won directly. This is not a coincidence: for every other position of the white bishop there exists more than one corresponding square, and moving the bishop to such a position is not the right way to challenge the defence. A complete list of all corresponding squares may be used for a a proof of assertion (2) above, but this plays no role for our analysis. Let us now prove assertion (1) for the corresponding squares in our table.
Trivially, d7 is the only square corresponding to d3. Next, assume that the white bishop is on g2. In order to keep it from d5, the black bishop must be on the diagonal a2–g8, but if it is not standing on f7, then White can win a pawn immediately. For instance, with the black bishop on c4 there follows 1. Bh3 Be6 2. Bf1 Bd7 3. Bd3.
With the white bishop on f1, it is clear that a corresponding square can only be c6 or e8. However, with the black bishop on c6 there follows 1. Be2 Be8 2. Bf3 Bf7 3. Bg2, and the black bishop must leave the corresponding square. This line also shows that c6 is the only square corresponding to e2.
With the white bishop on f3, c4 is clearly the only square where the black bishop covers d5, is ready to answer Bg2 by Bf7, and where it prevents the move Be2. If the black bishop were on a2, b3, e6 or g8, then 1. Be2 would win a pawn right away.
Finally, consider the white bishop on h3. The black bishop must be on d7 or g6, in order to protect the Pf5 and answer Bf1 by Be8. However, with the black bishop on d7 there follows 1. Bg2, and Black must let the bishop to d5, since 1. . . . Be6?! would lose a pawn after 2. Bf1 Bd7 3. Bd3.
Let us now turn to a detailed analysis of the ending, starting from diagram 2.
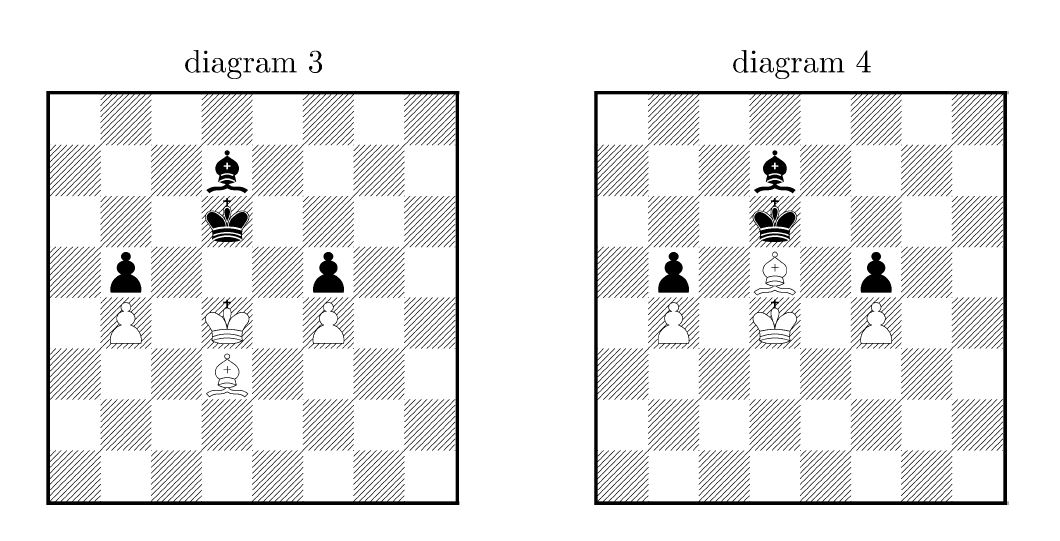
1. Bc8 Be4
2. Ke3
At this point White can make only make progress by means of a king manoevre, of course, since the bishop can only return to a6. The idea is simple: by making a little round tour and returning to d4 in an odd number of moves (namely five), White passes the move to Black, assuming that the black king moves between d6 and e7 so as to keep the white bishop trapped in the upper left region. At move 4 Black will also have the option of moving the king to d5, threatening to penetrate White’s camp, but here, too, he will be forced back to d6 immediately. Otherwise every other move by Black allows the white bishop to get free more quickly. At the moment Black cannot play 2. . . . Kd5?, of course, since the resulting pawn ending would be lost immediately (3. Bb7+ Kc4 4. B×e4 f×e4 5. f5 Kd5 6. f6 Ke6 7. K×e4 K×f6 8. Kd5).
Instead of the text move, 2. Kc3 would be less accurate, since after 2. . . . Ke7 3. Kd2 Kd6 White cannot continue 4. Ke2?? Kd5. Instead White can transpose to the main line by 4. Ke3.
2. . . . Ke7
3. Ke2 Kd6
4. Kd2 Kd5 4. . . . Ke7 makes no difference.
5. Kc3 Kd6
Obviously, any bishop move would be answered by 6. Bb7+, and 5. . . . Kc6 by 6. Be6.
6. Kd4 Kc7 After 6. . . . Bc2(b1) 7. Bb7 the white bishop also gets free, while other moves would even lose the Pf5 right away.
7. Be6 Kd6
8. Bb3 Bb7
Black already has to play accurately: the text move is the only one which forces White to go through Stage 2 of the winning procedure. After 8. . . . Bb1?! the white bishop can already occupy d5, while other moves along the diagonal a8–h1 allow White an immediate win of a pawn: 8. . . . Bc6?! 9. Bc2 Bd7 10. Bd3, or 8. . . . Bf3?! 9. Bc2 Bg4 10. Bd3.
9. Bc2 Bc8
10. Bd3 Bd7
Now position 3 has been reached. At this point we regard Stage 1 as finished, although the white bishop actually got free four moves ago.
11. Bf1 Be8
It was shown above that e8 is the only square corresponding to f1, and how White gets through Stage 2 after 11. . . . Bc6. Now a suitable moment has arrived for White to apply a king manoevre in the rear.
12. Kd3! Kd7
All things considered, this is the most tenacious defence for Black. There are numerous alternatives, but they all prove insufficient:
(a) After 12. . . . Bg6 or Bh5 there follows 13. Ke3 Be8 14. Kd4. White has successfully applied triangulation, and the black bishop must leave the corresponding square.
(b) After 12. . . . Bf7?! White can again proceed as in line (a), but in this case he can win even more quickly by 13. Bh3 Be6 (13. . . . Bg6? 14. Kd4 Bh7 15. Bf1) 14. Kd4 Bd7 15. Bg2, and Black must let the white bishop to d5, since 15. . . . Be6? 16. Bf1 Bd7 17. Bd3 would lose a pawn right away.
(c) After 12. . . . Bc6 13. Bh3 Be4(d7) 14. Kd4 the black bishop cannot reach the corresponding square g6.
(d) 12. . . . Bd7 is the most tenacious of the bishop moves, but again White answers 13. Bh3, intending to win with 14. Kd4 as in line (c). Black can prevent this only by 13. . . . Kd5, but after 14. Bg2+ Kd6 15. Kd4 he must let the white bishop to d5, as in line (c). If in plays 14. . . . Ke6 instead of 14. . . . Kd6, then the white bishop also reaches d5 after 15. Kd4.
(e) 12. . . . Kd5(c6) 13. Bg2+ Kd6?! 14. Bh3 loses a pawn after 14. . . . Bg6 15. Kd4 Bh7 16. Bf1 or 14. . . . Bd7 15. Kd4 Be6 16. Bf1 Bd7 17. Bd3. Therefore it is better for Black to move the king to a different square on move 13 (13. . . .Kd5–e6 or Kc6–c7), but this allows the white bishop again to reach d5.
(f) 12. . . . Ke6 is again answered by 13. Bg2, intending to get the bishop to d5 after 14. Kd4. Black can be prevent this only by 13. . . . Kd6, which leads to line (e), or by 13. . . . Bf7, which also loses a pawn after 14. Bc6. This is yet another example how a black king move to a white square may obstruct the bishop.
(g) 12. . . . Kc7 or 12. . . . Ke7 is answered by 13. Kc3!, putting Black in zugzwang. Black can no longer play 13. . . . Kd7, and after 13. . . . Kd6 14. Kd4 or 13. . . .Bd7(c6) 14. Kd4 Kd6 White has successfully applied triangulation: the black bishop must leave the corresponding square or has left it already. It remains to consider 13. . . . Kc7–c6 or 13. . . . Ke7–e6, but these moves lead to lines (e) or (f), respectively; here the fact that the white king is placed on c3 rather than d3 plays no role.
13. Be2
White takes advantage of the fact that the black bishop cannot move to the corresponding square c6.
13. . . .Kd6
This is now the only move which requires further consideration. In every other case there follows 14. Kd4, and after 14. . . . Kd6 the black bishop would be on the wrong square. Now, however, Black would be fine after 14. Kd4?! Bc6.
14. Kc3
Now White tries to apply triangulation, taking advantage of the fact that the black king has already returned to d6 in two moves. After 14. . . . Bc6 15. Kd4 or 14. . . . Kc6 15. Kd4 Kd6 the goal has been achieved: the black bishop has left or must leave the corresponding square.
14. . . . Bd7
Black tries to answer king triangulation by bishop triangulation: after 15. Kd4?!
Bc6 White would have to start over again. After 14. . . . Bc6 or any king move other than 14. . . . Kd5 there follows 15. Kd4, as shown in the preceding note. Thus it only remains to consider 14. . . . Kd5, which is answered by the obvious reply 15. Lf3+. Now 15. . . . Ke6 16. Kd4 allows the white bishop to reach d5, while after 15. . . . Kd6 16. Kd4 the black bishop cannot move to the corresponding square c4.
15. Bf1!
The bishop returns to f1 so as to frustrate bishop triangulation by Black. Now 15. . . . Bc6 is answered by 16. Bh3 Bd7(e4) 17. Kd4, and the black bishop cannot bet to g6.
15. . . . Kd5
This allows White to finish Stage 2 as in line (e) in the note on move 12. However, Black has no better alternative. After any other king move there follows 16. Kd4 Kd6 17. Bd3, and any bishop move other than 15. . . . Bc6 is likewise answered by 16. Kd4, whereupon the black bishop cannot move to e8.
16. Bg2+ Kd6
17. Kd4 Be8 17. . . . Be6?! 18. Bf1 Bd7 19. Bd3 would lose a pawn right away.
18. Bd5 Bd7
We have reached diagram 4, so Stage 3 of the winning process begins. As noted earlier, this stage was analyzed in detail by previous authors, so we only show one main line.
19. Bb3 Bc8
20. Bf7 Bb7
21. Be8 Ba6
22. Kd3 Ke7
23. Bc6 Kd6
24. Bf3 Bc8
25. Kd4 Bd7
26. Bd1 Be8
27. Bc2 Bd7
28. Bd3
The end of Stage 3 is reached, and Black cannot keep his pawns any longer. We only consider a line where Black gives up the pawn 5, for in the other case the winning procedure is similar.
28. . . . Be8
Of course 28. . . . Kc6 would be weaker, since after 29. Ke5 White gains not only a pawn but also an active king position.
29. B×f5 Bc6
30. Bd3 Bd7
31. f5 Bc6
32. Be2 Be8
33. Ke4 Bd7
34. Kf4 Be8
There was hardly any need to consider other options for Black during the preceding moves: clearly White can always move the king to g5, threatening to support his passed pawn, which ensures an easy win unless Black can get his king to f8 in time. The only thing White has to watch out for is a counterattack against the Pb4, and indeed, after 34. . . . Kd5 (instead of 34. . . . Be8) Black threatens 35. . . . B×f5 36. K×f5 Kd4, eliminating White’s last pawn. However, in this case there follows 35. f6, when 35. . . . Ke6 36. Bg4+ or 35. . . . Be8 36. B×b5 loses immediately, while 35. . . . Kd6 36. Kg5 is also hopeless.
35. Bf1
This is a waiting move: after 35. Kg5 Ke7 White finds it more difficult to make further progress. In this case 36. f6+ Kf8 leads to the kind of position which White wants to avoid: he cannot win the Pb5 without giving up the Pf6, which is already advance to far, and minor details decide about win or draw. After the text-move a counterattack is again insufficient for Black, for instance 35. . . . Kd5 36. Kg5 Kd4 37. f6 Kc3 38. B×b5 Bf7 39. Be8 B×e8 40. b5, and one of the white pawns will promote. This line shows why White played 35. Bf1 rather than 35. Bd3. The bishop sacrifice 39. Be8 is not the only way to win, but the quickest one. If in this line Black plays 36. . . . Ke5 (instead of 36. . . . Kd4), then there follows 37. f6 Ke6 38. Bd3! Kd6 39. Kf5, and Black no longer has any reasonable move.
35. . . . Ke7
Black’s retreats the king and gives up the square e5, but this is now his best course. The alternative 35. . . . Bd7?! also does not prevent White from breaking through with the king to the Pb5, this time by threatening to support his passed pawn: 36. Kg5 Ke7 37. Kg6 Kf8 38. Kf6. With the king marching along the sixth rank, the win is straightforward: 38. . . . Be8 39. Ke6 Bc6 40. Bd3 Be8 41. Kd6 Kf7
42. Be4 Kf6. In the main line this position will be reached two moves later, and the win require one more slight subtlety.
36. Ke5 Bd7
37. Bd3 Bc6
38. Be4 Bd7
39. . . . Be8 40. Kd5 leads to the position which is reached three moves later in the main line.
39. Kd5 Be8
Now Black has reached the best defensive setup against the current arrangement of White’s pieces. White has to pass the move to Black by means of triangulation with the bishop.
40. Bd3 Bd7
After 40. . . . Kf6 White can transpose to the main line by 41. Kc5, when Black must reply 41. . . . Bd7, but slightly quicker is 41. Kd6 Bh5 42. B×b5 K×f5 43. Bd7+ or 41. . . . Kf7 42. Be4 Kf6 43. Bc6. In both cases the main line is again reached, but play is shortened by two or four moves, respectively.
41. Bc2 Be8
Again Black has nothing better: 41. . . . Kf6 42. Kd6 Be8 43. Bd3 or 41. . . . Kf7 42. Kd6 Be8 43. Be4 leads to the preceding note.
42. Be4 Bd7
After 42. . . . Kf6 43. Kc5 Bd7 just leads to a transposition of moves, while after 43. . . . Ke5 44. Bc6 Bh5 45. Bd7! White wins the Bb5 without giving up the Pf5. However, in the latter line White must avoid 45. B×b5? K×f5 46. Bd7+ Kf6, and even though White advance his last pawn, Black draws. The reason is that the white king is not placed well enough on c5; with the king on d6 White would win easily, as it ultimately happens in the main line.
43. Kc5 Kf6
Black is again in zugzwang. Other king moves are also answered by 44. Kd6, followed by Bc6 or f6, while after 43. . . . Be8 there follows of course 44. Bc6.
44. Kd6 Be8
Or 44. . . . Bc8 45. Kc6 etc.
45. Bc6 Bh5
46. B×b5 Kxf5
47. Bd7+
No further analysis is needed: by pushing the pawn White wins without any further problem.





Kommentare
Gratulation an diese Entdeckung, die vermutlich spätestens nach Vorliegen der 8-Steiner bestätigt wird!
Alle Kommentare dieses Beitrages als RSS-Feed.